Here is a good explanation of thermodynamics of open systems, plus the valid methodology used to the create the Etp model:
http://chemwiki.ucdavis.edu/Physical_Chemistry/Thermodynamics/A_System_And_Its_Surroundings
A System and Its Surroundings
In thermodynamics, it is imperative to define a system and its surroundings because that concept becomes the basis for many types of descriptions and calculations.
Introduction
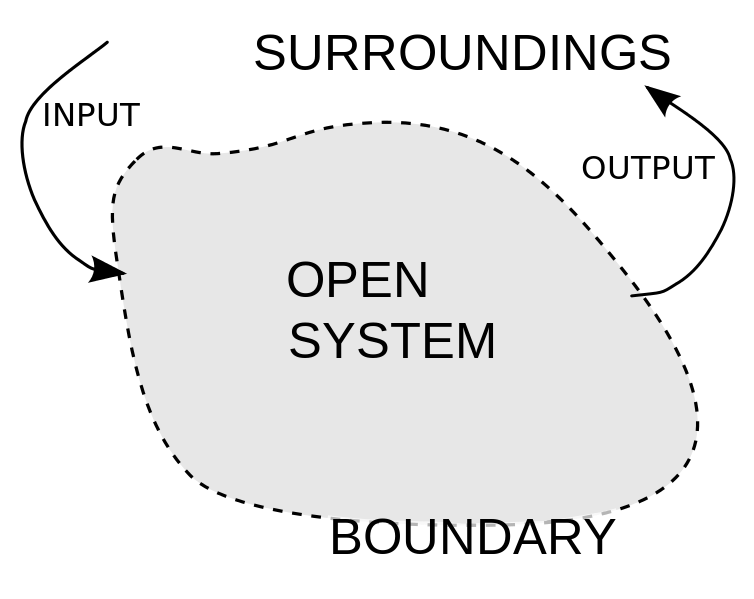
A primary goal of the study of thermochemistry is to determine the quantity of heat exchanged between a system and its surroundings. The system is the part of the universe being studied, while the surroundings are the rest of the universe that interacts with the system. A system and its surroundings can be as large as the rain forests in South America or as small as the contents of a beaker in a chemistry laboratory. The type of system one is dealing with can have very important implications in chemistry because the type of system dictates certain conditions and laws of thermodynamics associated with that system.
Open System
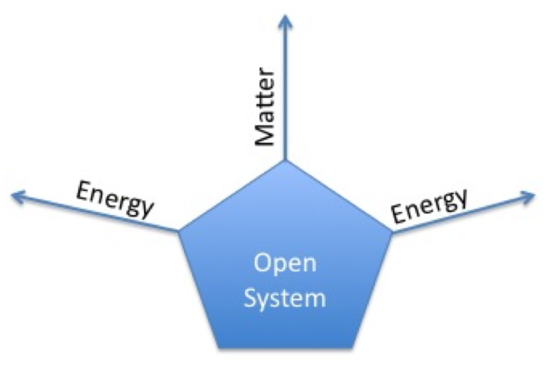
An open system is a system that freely exchanges energy and matter with its surroundings. For instance, when you are boiling soup in an open saucepan on a stove, energy and matter are being transferred to the surroundings through steam. The saucepan is an open system because it allows for the transfer of matter (for example adding spices in the saucepan) and for the transfer of energy (for example heating the saucepan and allowing steam to leave the saucepan).
Let us examine how matter and energy are exchanged in an open system. Matter can be exchanged rather easily: by adding matter (i.e spices) or removing matter (i.e tasting what is being cooked). Energy exchange is a little bit more complicated than matter exchange. There are a couple of ways energy can be exchanged: through heat and through work (a more in-depth discussion of heat and work has been included below). Energy induced through heat can be demonstrated by bringing the system close to an object that dissipates heat (i.e. Bunsen burner, stove, etc.). By doing so, one is able to change the temperature of the system and therefore, induce energy through heat. Another way to increase the energy is through work. An example of inducing work is by taking a stirrer and then mixing the coffee in the cup with the stirrer. By mixing coffee, work is done as the coffee is being moved against a force.
Note: the blue diagram depicting the transfer of energy and matter is showing how energy and matter can enter the system AND leave the system. Do not be fooled by the one way arrows.
https://en.wikipedia.org/wiki/Thermodynamic_system
Open system
In an
open system, matter may flow in and out of some segments of the system boundaries. There may be other segments of the system boundaries that pass heat or work but not matter. Respective account is kept of the transfers of energy across those and any other several boundary segments.
The region of space enclosed by open system boundaries is usually called a
control volume.
Here is a description of the boundary conditions used in the Etp model and the derivation of equation#7 from the
Entropy Rate Balance Equation for Control Volumes:
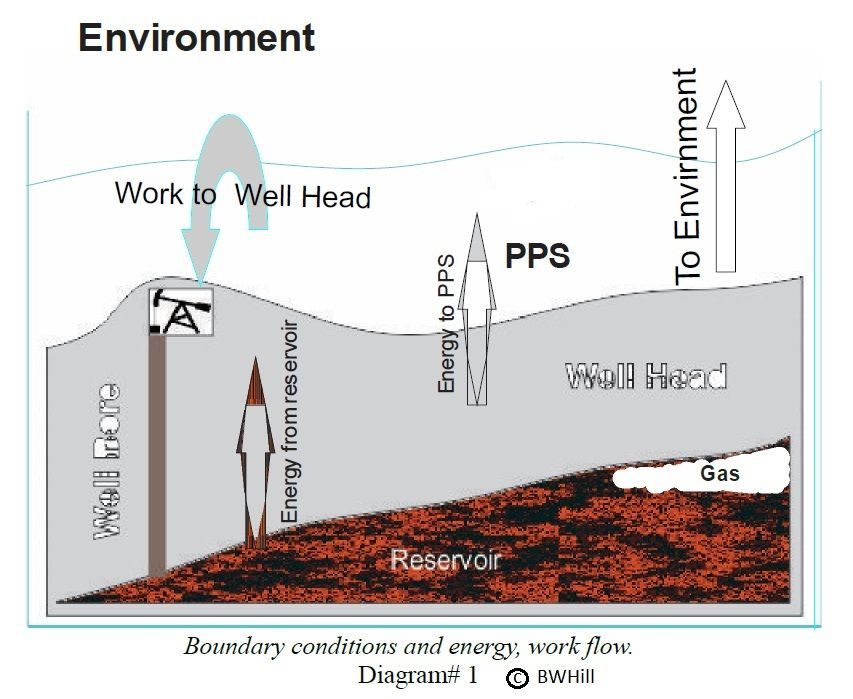
"Crude oil is used primarily as an energy source; its other uses have only minor commercial value. To be an energy source it must therefore be capable of delivering sufficient energy to support its own production process (extraction, processing and distribution); otherwise it would become an energy sink, as opposed to a source. The Total Production Energy ($$E_{TP}$$) must therefore be equal to, or less than EG, its specific exergy. To determine values for $$E_{TP}$$ the total crude oil production system is analyzed by defining it as three nested Control Volumes within the environment. The three Control Volumes (
where a control volume differs from a closed system because it allows energy and mass to pass through it's boundaries) are the reservoir, the well head, and the Petroleum Production System (PPS). The PPS is where the energy that comes from the well head is converted into the work required to extract the oil. The PPS is an area which is distributed within, and throughout the environment. It is where the goods and services needed for the production process originate. This boundary make-up allows other energy, and mass transfers to be considered as exchanges, such as natural gas used in refining, electricity used in well pumping, or water used for reservoir injection."
~BW Hill
Values for $$E_{TP}$$ are derived from the solution of the
Second Law statement, the
Entropy Rate Balance Equation for Control Volumes:
$$\frac{dS_{CV}}{dt}
=\sum_j\frac{\dot{Q}_{j}}{T_{j}}
+\sum_i\dot{m}_{i}s_{i}
-\sum_e\dot{m}_{e}s_{e}
+\dot{\sigma}_{cv}$$
"Where $$\frac{dS_{CV}}{dt}$$ represents the time rate of change of entropy within the control volume. The terms $$\dot{m}_{i}s_{i}$$ and $$\dot{m}_{e}s_{e}$$ account, respectively, for rates of entropy transfer into and out of the control volume accompanying mass flow. The term $$\dot{Q}_{j}$$ represents the time rate of heat transfer at the location on the boundary where the instantaneous temperature is $$T_{j}$$. The ratio $$\frac{\dot{Q}_j}{T_j}$$ accounts for the accompanying rate of entropy transfer. The term $$\dot{\sigma}_{cv}$$ denotes the time rate of entropy production due to irreversibilities within the control volume."
~(Taken from
Fundamentals of Engineering Thermodynamics by Moran and Shapiro)
Because there is only one temperature boundary (at the exit point of the reservoir) and no crude oil enters the reservoir from the environment, the equation reduces to:
$$\frac{dS_{CV}}{dt}=\frac{\dot{Q}_{j}}{T_{j}}-\dot{m}_{e}s_{e}+\dot{\sigma}_{cv}$$
giving: $$\frac{BTU}{sec*°R}$$
For this application, crude oil and water can be treated as incompressible substances. Their specific entropies are only affected by a temperature change.
For specific heats: $$c_{v}=c_{p}=c$$, and $$s_{2}-s_{1}=c*\ln{\frac{T_{2}}{T_{1}}}$$ The reservoir temperature is constant, therefore the entropy of the reservoir must decrease at the same rate that the entropy is transferred from the reservoir by mass flow. Thus, the heat leaving the reservoir is negative in sign and the equation becomes:
$$\frac{\dot{Q}_{j}}{T_{j}}=\dot{\sigma}_{cv}$$
giving: $$\frac{BTU}{sec*°R}$$
The rate of entropy production in the petroleum production system is equal to the rate of heat extracted from the reservoir divided by the reservoir temperature.
The rate of irreversibility production in the petroleum production system therefore becomes:
$$\dot{I_{cv}}=T_{O}*\dot\sigma_{cv}$$
giving: $$\frac{BTU}{sec}$$
Where $$T_{O}$$ equals the standard reference temperature of the environment, 537 °R (77° F).
Therefore:
$$E_{TP}=\int_{t1}^{t2}\dot{I_{cv}}dt$$
giving: $$BTU$$
Because the mass removed from the reservoir is limited to crude oil and water, the increase in $$E_{TP}$$ per billion barrels (Gb) of crude extracted as $$ds=c\frac{dT}{T}$$ is:
(
Equation#7)
$$\frac{E_{TP/lb}}{Gb}
=\begin{bmatrix}\frac{(m_{c}*c_{c}
+m_{w}*c_{w})(T_{R}-T_{O})}{m_{c}} \end{bmatrix}/Gb$$
giving:
BTU/lb/Gb
$$m_{c}$$ = mass of crude, lbs.
$$c_{c}$$ = specific heat of crude, BTU/lb °R
$$m_{w}$$ = mass of water, lbs.
$$c_{w}$$ = specific heat of water, BTU/lb °R
$$T_{R}$$ = reserve temperature, °R
$$T_{O}$$ = standard reference temperature of the environment, 537 °R
$$s_{i}$$ = specific entropy into the control volume
$$s_{e}$$ = specific entropy exiting the control volume
BTU/gal/Gb for 35.7° API crude = BTU/lb/Gb * 7.0479 lb/gal
Evaluation of $$E_{TP}$$ from
Equation# 7 requires the determination of three variables: mass of the crude ($$m_{c}$$) mass of the water ($$m_{w}$$), and the temperature of the reservoir ($$T_{R}$$). These must be determined at time (t).
1) The mass of crude at time (t) is derived from the cumulative production function,
2) the mass of water is derived from the average % surface water cut (fw) of the reservoir,
3) temperature of the reserve is derived from the well depth. This assumes an earth temperature gradient of 1°F increase per 70 feet of depth.
-------------------------------
What exactly do you find wrong with the methodology, above, used to develop the Etp function?
---Futilitist