Origin and verification of e=(Th-Tc)/Th

Tom Booth said:
BTW, I never had any desire or intent to "challenge thermodynamics".

The experiments were, as I explained, intended to demonstrate the fact that a Stirling engine would fail to operate if the flow of heat through to the "cold reservoir" were blocked or interrupted.

I've also done similar experiments with high temperature engines and a propane torch as the heat source with the same results.

A propane torch burns at around 2000°F.

Your opinion that there is simply too little heat to possibly be measurable is just plain wrong as well as irrational.
Click to expand...
Then explain why it is wrong. You admit you have not measured the heat flow in, or the heat flow out, or the work done.

I have estimated the work done (tiny) and pointed out how little heat flow would be needed to support that, which would explain why you can’t detect a temperature rise due to heat flow.

You have offered nothing to suggest a flaw in this reasoning. So on what basis do you state I am “plain wrong”?
 
exchemist said:
Then explain why it is wrong. You admit you have not measured the heat flow in, or the heat flow out, or the work done.

I have estimated the work done (tiny) and pointed out how little heat flow would be needed to support that, which would explain why you can’t detect a temperature rise due to heat flow.

You have offered nothing to suggest a flaw in this reasoning. So on what basis do you state I am “plain wrong”?
Click to expand...
Maybe you can explain what might possibly prevent a steady heat applied for two or three hours to a 2 millimeter thick aluminum plate from passing through the plate and into the engine. The air inside the engine on the other side of the plate, the entire time being agitated by the displacer, forcing the air against the plate to heat it each cycle.

Your calculations are mere guesswork. The thermal image camera temperature readings clearly show the upper inside area of the engine becoming quite hot under the insulation.

The forum will not let me upload this image but I can post a link:


Pulling down the insulation, the thermal image shows 87°F for the lower part of the engine and 62°F for the upper part.

The hot water was still about 160°F under the engine, and the room temperature is 64°F

The above image is a screenshot from this video:


At that point the engine had been running on hot water for about 2 hours already which was refreshed about every 20 minutes with fresh boiling water from the tea kettle.

I ran the experiment for about another hour and there was still no change.

The upper side of the engine stayed about room temperature or slightly lower.

The upper side of the engine under the insulation was even colder. A few degrees below room temperature.

The lower inside temperature was 87°F
 
Tom Booth said:
Maybe you can explain what might possibly prevent a steady heat applied for two or three hours to a 2 millimeter thick aluminum plate from passing through the plate and into the engine. The air inside the engine on the other side of the plate, the entire time being agitated by the displacer, forcing the air against the plate to heat it each cycle.

Your calculations are mere guesswork. The thermal image camera temperature readings clearly show the upper inside area of the engine becoming quite hot under the insulation.

The forum will not let me upload this image but I can post a link:


Pulling down the insulation, the thermal image shows 87°F for the lower part of the engine and 62°F for the upper part.

The hot water was still about 160°F under the engine, and the room temperature is 64°F

The above image is a screenshot from this video:


At that point the engine had been running on hot water for about 2 hours already which was refreshed about every 20 minutes with fresh boiling water from the tea kettle.

I ran the experiment for about another hour and there was still no change.

The upper side of the engine stayed about room temperature or slightly lower.

The upper side of the engine under the insulation was even colder. A few degrees below room temperature.

The lower inside temperature was 87°F
Click to expand...
If, as you appear to concede, the engine is doing no work other than to overcome frictional losses, then the heat it requires will be minute. You have no hope of detecting it. It will be lost in the “noise” and margin of error of your temperature measurements.
 
exchemist said:
If, as you appear to concede, the engine is doing no work other than to overcome frictional losses, then the heat it requires will be minute. You have no hope of detecting it. It will be lost in the “noise” and margin of error of your temperature measurements.
Click to expand...
Right, so you have expressed your opinion.

The distance between the top and bottom plates, (heat exchangers) is less than 1 inch, with a displacer inside fanning the air up and down between the two.

The bottom plate is over 100°F, probably closer to 200°F most of the time. The top plate is in contact with ambient air at about 65°F

Yet the top side of the engine, inside and out gets colder, the temperature falling to somewhere between 62°F and 64°F according to actual thermal image temperature readings, but you still think there is nothing contrary to the Carnot concept or formula, that 5 times MORE heat is supposed to be going to the top cold side than is used to run the engine?

How about taking another look.

Do you notice that in the video there is a red "hot spot" at the power piston, in the middle of the otherwise cold top side?

That is due to the friction from the piston moving up and down.

That represents "work" that the working fluid is doing. The work involved in driving the piston and driving the engine generating friction.

No, it isn't particularly "useful work" but it is work performed by the "working fluid".

So, the top of the engine in general, according to the Carnot limit theory should be receiving 5 times more heat than the energy that went into producing that friction, which is obviously not no work at all, or negligible.. The heat from friction generated by the work output at the power piston is clearly visible, and yet the "sink" side of the engine is otherwise colder than the ambient surroundings.

Your just exercising willful blindness big time. So absolutely brainwashed by your "education" you can't see the obvious when it's right in front of your eyes.

I guess a Stirling engine is some kind of super power insulation.

What's the R value of less than 1 inch of "insulation" when there is LESS than NO heat at all getting through?

Anyway, no point in further discussion or debate on that issue, as you obviously have no common sense whatsoever and are just going to adhere to what you believe regardless of any evidence to the contrary.
 
exchemist said:
Then explain why it is wrong. You admit you have not measured the heat flow in, or the heat flow out, or the work done.

...
Click to expand...
There is no such thing as "heat flow". Measuring "heat flow" is not possible.

Heat is kinetic energy. For a gas, the measure of its kinetic energy is the temperature.
 
exchemist said:

Rate of heat flow - Wikipedia

en.wikipedia.org en.wikipedia.org
Click to expand...
And as your reference states, the so-called "heat flow" depends on the ∆T and:

"heat flows from the side at higher temperature to the one at lower temperature, not the other way around."

So which way would the heat "flow" from the working fluid or cold plate at 62° and 64° F respectively, and the ambient surroundings at about 65°F minimum. All as measured repeatedly over the course of a few hours?

The significant observation is that any "flow" would be opposite in direction to what is predicted or assumed or calculated by the Carnot theory / formula.

Maybe one or two experiments could be attributed to instrument failure, "margin of error" or whatever, but this has been dozens and dozens of experiments, conducted in many different ways, using all kinds of thermal measuring instrumentation over the course of several years.

The cumulative evidence indicates the Carnot efficiency limit equation has no basis in fact. It cannot be demonstrated experimentally.

Even if the temperature readings are a little off they would have to be off by a factor of at least five, and simultaneously take readings that are both higher and lower than predicted by the Carnot assumption. i.e. the heat from friction from work at the power piston reading too high while the top of the engine reads too low, all in the same thermal image recording.

And the supposed "error" is always contradictory to what the Carnot predicted values should be for every experiment for dozens of experiments conducted at different times.

If you want to calculate the exact flow, or lack of flow, anyone can do so based on the temperature readings.

Personally I don't see any point in determining exactly since the readings are infact opposite to the whole Carnot theory.

How much "waste heat" is "flowing" from an object (top plate) at 62°F to an object (ambient air) at 65°F ?

The only way to determine that is by the temperature readings, as your reference shows. And the fact that heat can only "flow" or transfer from a higher to a lower temperature object or substance, not the other way around.

So yes, the work output as well as the heat flow can be deduced from the recorded information. The thermal temperature readings.

It can be clearly seen that the work output (heat from friction at the power piston showing up red) greatly exceeds the "waste heat" at the top plate showing up dark blue)

You have both the visual, color indicators as well as the digital readout, and also the little green and red squares dancing around tracking the hottest "Max". and coldest "Min". objects in the view screen.

Almost the entire time the green square indicating the coldest point stays locked in on the top of the engine and the red on the power piston.

I don't see how that is explainable in terms of the Carnot efficiency assumptions.


Please provide a reference to any comparable experiment that actually does validate the Carnot limit assumptions. Here is your opportunity to prove me wrong. Please provide the empirical, experimental evidence. Yours or anyone else's in however long this Carnot theory has been around.
 
Anyway, rather than ignore or dismiss, or try to explain away what seems to me clear experimental results, I studied the Stirling engine in more depth.

To make a long story short, I've reached the conclusion that the Stirling Cycle is actually very similar to the Vuilleumier cycle. Using high heat at one end to produce a cooling effect at the other.

A Vuilleumier cycle "runs on" heat to transfer heat. In other words a heat driven heat pump.

The Vuilleumier takes heat in at both ends and moves the heat into the central area.

The difference is, the Stirling engine uses high heat at one end as the means for drawing in additional heat at the other end, but instead of just moving the heat, the heat is converted into mechanical motion or "work".

Instead of moving the heat as in a Vuilleumier cycle the Stirling engine converts heat into work.

Otherwise the Stirling and Vuilleumier cycle are practically identical.

en.m.wikipedia.org

Vuilleumier cycle - Wikipedia

en.m.wikipedia.org en.m.wikipedia.org
 
Tom, ExChemist, has answered your question quite politely. The Carnot Theorem was developed by Carnot, Clausius, and Kelvin. Your request to nail down an exact source, is a little like trying to nail down whom first flew. Go ahead and search 'who flew first' and see if you get a single answer. Many have derived, refined, and named, the Carnot theorem since.

Your point of efficiency being determinable from Qc is answerable by the following questions:

100 J in, 1 J of Work output, 10 J heat rejected. What is the efficiency?

100 J in, 0.1 J of Work output, 1 J heat rejected. What is the efficiency?

100 J in, 0.1 J of Work output, 0.0 J heat rejected. What is the efficiency?

Answers in order:

1%, measured heat rejected error.

0.1%, measured heat rejected error.

0.1%, measured heat rejected error.

What's the moral of the story? Efficiency is not dependent on heat rejected measurements.
 
Fool said:
Tom, ExChemist, has answered your question quite politely. The Carnot Theorem was developed by Carnot, Clausius, and Kelvin. Your request to nail down an exact source, is a little like trying to nail down whom first flew. Go ahead and search 'who flew first' and see if you get a single answer. Many have derived, refined, and named, the Carnot theorem since.

Your point of efficiency being determinable from Qc is answerable by the following questions:

100 J in, 1 J of Work output, 10 J heat rejected. What is the efficiency?

100 J in, 0.1 J of Work output, 1 J heat rejected. What is the efficiency?

100 J in, 0.1 J of Work output, 0.0 J heat rejected. What is the efficiency?

Answers in order:

1%, measured heat rejected error.

0.1%, measured heat rejected error.

0.1%, measured heat rejected error.

What's the moral of the story? Efficiency is not dependent on heat rejected measurements.
Click to expand...
Quite so.

There is a history to this poster that may be relevant. He seems to be taken with an idea of Tesla (yes, him) at the turn of the last century that one could run an engine on ambient heat only. This was the article he referred to, written by Tesla, which from a historical point of view is an interesting curiosity:
https://www.unz.com/print/Century-1900jun-00175. It covers all manner of things but there is a section towards the end headed "First Efforts to Build the Self-Acting Engine.....".

Tom thinks, or suspects, (or at any rate he did when he posted about this on another forum 3 years ago) that, as he can't detect any increase in temperature of the heat sink end of his model Stirling engine, the Carnot theorem may not apply to the Stirling cycle and that it may be able to convert heat to work without rejecting any waste heat.

There was even the start of an attempt to crowd-fund a project to explore Tesla's idea: https://experiment.com/projects/hohohltuqpivlpspyewk/methods , though this has not progressed.

Tom has in the past devoted considerable effort to rubbishing Carnot, on the basis of his use of the flawed c.18th concept of "caloric". His repeated demands, in the present thread, for the original source of the Carnot Cycle efficiency formula betray a wish to cast doubt on that as well.

While perpetual motion "free energy" devices of the 1st kind are two a penny on science forums, this is only the second time* I've come across a believer in perpetual motion of the 2nd kind. So that makes Tom an interesting character.


* The other time was a Japanese (I think) who thought an IR photovoltaic cell could be used to cool a fridge and run a light bulb as it did so.
 
Last edited:
exchemist said:
It comes I think from Clausius inequality: https://en.wikipedia.org/wiki/Clausius_theorem

(...)

Basically Clausius showed that for the Carnot cycle, Qh/Th + Qc/Tc =0 cf. https://www.ncbi.nlm.nih.gov/pmc/articles/PMC7516509/
N.B. he treats Qc as -ve, as it is heat leaving rather than entering the cycle.

So we have Qh/Th = -Qc/Tc, which can be rearranged as Qh/Qc = - Th/Tc

Using Clausius's sign convention, the efficiency , η = 1+ Qc/Qh (+ve because Qc is a -ve quantity in his convention).

So therefore η = 1- Tc/Th, or η = (Th-Tc)/Th for the Carnot cycle. This means η < (Th-Tc)/Th for all real engines.

(...)
Click to expand...

The Clausius inequality seems a bit problematic if you try to plug some actual real values into it

Qh/Th + Qc/Tc = 0

Let's say 100 joules in

Qh = 100J

At temperature 375 K for example

Th = 375

Let's say Qc = 50 for now

Tc = 300K ambient

Qh/Th + Qc/Tc = 0 ?
100/375 + (-)50/300 = 0 ?
.2666... - .1666... = .1

The only way it seems to actually work out to be true is where Qh/Qc is exactly proportional to Th/Tc

This was derived, though, based on a "Carnot engine" based on Caloric theory using some high level abstract Calculous.

In caloric theory temperature is the measure of a fluid; caloric, which today, we now know doesn't actually exist. Naturally, if temperature is a measure of a fluid, the measurement must be proportional to the quantity of fluid. Not so if we are only talking about one form of energy which is not necessarily conserved in that form.

The Carnot engine as originally conceived by Carnot depended on Caloric theory where all of the caloric, a fluid, passes through the engine.

There was no concept of heat conversion into work.

So, there are a number of potential problems and issues in bridging the gap between the old Caloric theory and the current modern view of heat as energy transfer


At any rate it seems that the Clausius inequality is based on an impossible engine that operated in an impossible way with zero efficiency by the modern view of heat as energy that is converted to work rather than ALL the heat "fluid" passing through like water through a turbine.

So we have the so-called "Carnot efficiency limit" equation emerging from this general confusion and conceptual uncertainty regarding the actual nature of heat.

At face value, (however it was derived), the Carnot limit formula is simply a temperature ratio. The ∆T itself.

An abstract mathematical formula derived from calculations based on a hypothetical but impossible engine that operated by means of the "transport" through, rather than the conversion, within, of a non-existent "caloric".

And none of this was ever subject to any kind of experimental validation whatsoever?

And we are not allowed to question any of this in any way without being labeled a "free energy crank"?

Personally I think it is way beyond the point where this should be put to some kind of empirical testing.

The kitchen table experiments I'm fumbling around trying to do with my limited skills and resources should have been done at least a century ago before accepting some simplistic temperature ratio as an absolute "LAW" controlling heat engine efficiency applicable to every engine that has or will ever be invented.
 
No need to explain all that to "fool". He has been stalking me across the internet for a long time.

So, exchemist, since you appear to be in agreement with the "fool" do you deny the fact that his examples violate conservation of energy?

Don't joules in need to equal joules (work + heat) out?
 
Tom Booth : would be really helpful to readers if you would use the quote function with greater judiciousness, so we can tell exactly what passage, and by whom, you are responding.
 
exchemist said:
(...)
Click to expand...

exchemist said:
". His repeated demands, in the present thread, for the original source of the Carnot Cycle efficiency formula betray a wish to cast doubt on that as well.

While perpetual motion "free energy" devices of the 1st kind are two a penny on science forums, this is only the second time* I've come across a believer in perpetual motion of the 2nd kind. So that makes Tom an interesting character.


* The other time was a Japanese (I think) who thought an IR photovoltaic cell could be used to cool a fridge and run a light bulb as it did so.
Click to expand...
Your portrayal of me as "demanding" anything is not accurate. It's a simple request for information I was never able to locate myself after a rather diligent search.

I'm also not a "believer" in any one view over the other when it came to the views of Kelvin vs Tesla on the subject of heat engines.

My stance was, and still is, completely agnostic. To start out with, I knew nothing of Carnot, Tesla or the second law or the Carnot efficiency limit I'm only a simple engine mechanic by trade. In general I know how engines work more by hands in experience, not theory

To me the debate was a debate between scientists to which I was completely indifferent.

I was simply interested in how Stirling engines work, as I was interested in building one as a matter of survival, living on some land off-grid.

So my approach was objective.

I thought both Carnot/Kelvin made a good argument, but Tesla had some good points also, and in my opinion, looking at all the evidence, the debate seemed as yet unresolved in any conclusive way.

I could find no reference to any actual experiments that settled the question one way or the other, aside from Joule, which did not address the issue of any "limit" on efficiency.

So, giving it some thought, I conceived of the experiments which I conducted to help settle the question in my own mind which might then help to guide my efforts at constructing a Stirling engine.

So far, objectively speaking, the results of my experiments appear to be in favor of Tesla's viewpoint, that there is no limit on the conversion of heat into work.

If, incidentally, that suggests some sort of "free energy" from ambient heat might be possible as Tesla believed is really neither here nor there.

My objective was simply to understand how a Stirling engine actually operates. I did not bring any preconceived notions to the table and I did not care one way or the other what might turn out to be the truth.

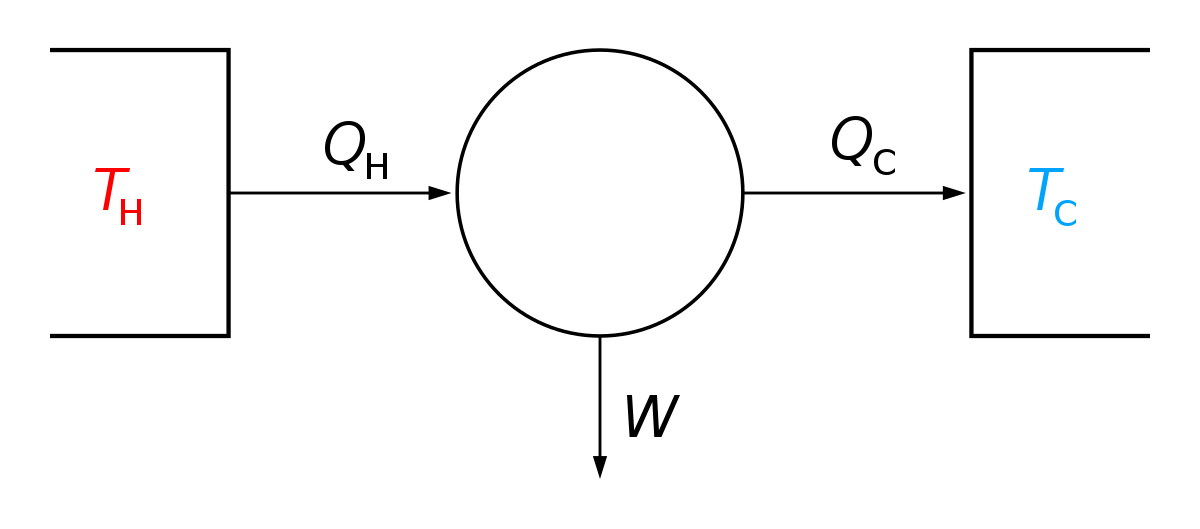
So I never set out with any intent to "cast doubt" on Carnot's assumptions, as I've said over and over, my experiments were originally intended to demonstrate how a Stirling engine would not operate if it were rendered unable to "reject" the excess "waste heat".

Well, I tried blocking this "rejection" in every possible way imaginable, with no effect.

From there I set about actually trying to measure the "waste heat" flow out of the engine, and could not detect any.

There was no intention on my part to produce any particular experimental results, one way or the other.

So your constant attempts at characterizing me as a "free energy crank" are not accurate.

I'm simply taking an objective stance. If there is an actual limit to the conversion rate as Carnot postulated, great, then I could work with that.

If on the other hand, Tesla was correct, then I could work with that

My only interest has been in obtaining accurate and reliable information that I could have in mind as a guide for designing my own engine.

As I mentioned before, assuming you need to make a provision for discarding "waste heat" if actually unnecessary is just throwing away "fuel". The heat that is actually necessary to operate the engine, so if you design the engine to discard 80% of the input heat as "waste heat" you are going to limit the engine to 20% efficiency by design
 
DaveC426913 said:
Tom Booth : would be really helpful to readers if you would use the quote function with greater judiciousness, so we can tell exactly what passage, and by whom, you are responding.
Click to expand...
Looking back on my recent posts, it appears I have:

So, I really don't know what you mean. All my posts appear to quote the relevant passages from whomever I happen to be responding to. I think?

Well, I see one exception. Exchemist long post about me to our new member "fool".

I thought I that instance reproducing exchemist entire post would be redundant.

Anyway, sure, if you like. This forum hides long quotes, which I'm not used to, so redundancy is not as much of an issue as on other forums where it can be unnecessarily repetitious and annoying to have to scroll through
 
Last edited:
Tom Booth said:
Looking back on my recent posts, it appears I have:

So, I really don't know what you mean. All my posts appear to quote the relevant passages from whomever I happen to be responding to. I think?
Click to expand...
It was just 73 and 74 in this page. Turns out 72 did have an attribution, to Exch, just that it was a lot of cumulative scrolling to find one.

(I thought maybe there were a few more earlier in the thread, but no matter. )

Tom Booth said:
This forum hides long quotes, which I'm not used to, so redundancy is not as much of an issue
Click to expand...
For my part, I make great effort to include only those specific parts I am quoting. I prefer to make it as easy as possible for readers to follow me.
 
Last edited:
DaveC426913 said:
Tom Booth : would be really helpful to readers if you would use the quote function with greater judiciousness, so we can tell exactly what passage, and by whom, you are responding.
Click to expand...
It would be helpful if you quoted the post of mine you had an issue with, so I could have at least one example of what you are complaining about, but you didn't do that either.

Do you have anything to add that actually relates to the thread topic?
 
DaveC426913 said:
It was just 73 and 74 in this page.
Click to expand...
I mention "you both" and also exchemist and fool, the only other participants in this discussion of late.

If you had been following a long with the conversation here, I don't see how anyone could miss that fact.

Regardless, thanks for pointing that out, I'll try to be more judicious in my use of the quote feature to save latecomers to the conversation having to bother looking back.
 
You must log in or register to reply here.
Back
Top