["Tom Booth"]
["Fool"]
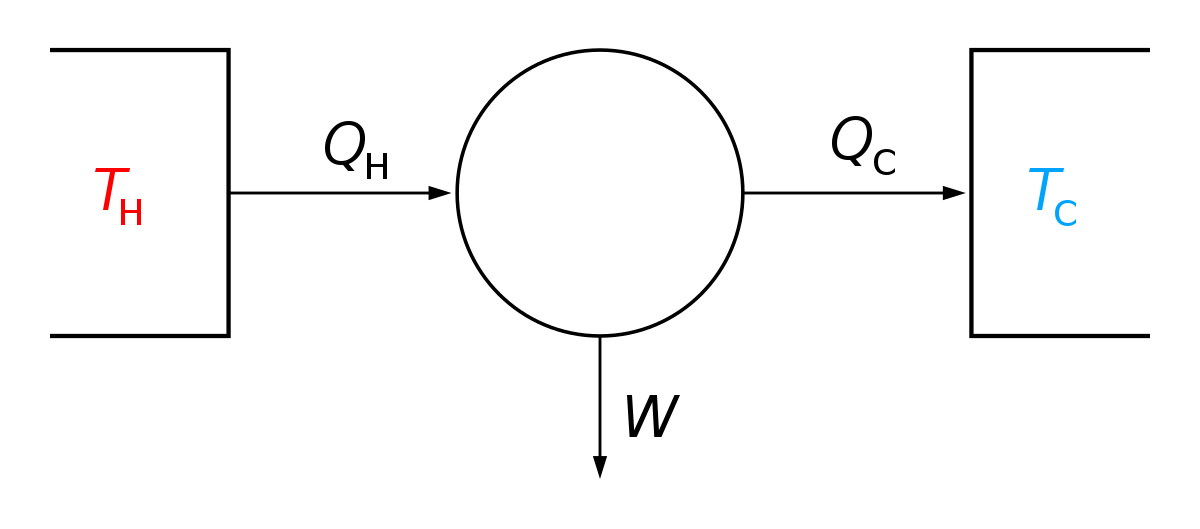
["Tom Booth"]The "first law" thermal efficiency equation is:
Qh=Ql+W
Which I don't have a problem with. It does not imply that work can exceed the heat input, but neither does it suggest that the work output in Joules cannot match the heat input in Joules.
The first law does not forbid the existence of an engine more efficient than the Carnot limit, not at all.["/Tom Booth]
Qh = Qc + W
Or rearranged:
W = Qh - Qc
Second Law:
Efficiency ratio:
n = W/Qh
Or combining first and second
n = (Qh-Qc)/Qh = 1- Qc/Qh
Qh and Qc have a linear relationship to Temperature. Meaning add 100 Joules get 100 K temperature increase. Add 200 Joules get 200 degrees increase.
So they are of the following form:
Qh = Th x Cv + K
Qc = Tc x Cv + K
Y intercept, constant: K = zero because, at absolute zero Kelvin, there will be no heat. Qh and Qc will be zero at zero Kelvin Temperature.
So those two simplify to:
Qh= CvTh
Qc=CvTc
Putting those into the previous equation for n, efficiency:
n = 1 - Qc/Qh
Or
n = 1 - (CvTc)/(CvTh)
The Cv's cancel, becoming one, which leaves:
n = 1- Tc/Th
That is Carnot's Theorem derived from the first and second laws. The first law, conservation of energy, manifests itself into a great many following areas. Maximum efficiency is just one of those.
Here is a more rigorous proof:
Relationship to ideal gas law:

en.m.wikipedia.org
Scroll down to the appropriate section. It uses a slightly higher level of mathematics.
There are other more complicated proofs should you care for them. It has been proven many different ways.
The Carnot Theorem is just for thermodynamic efficiency. Kinematic efficiency reduces the efficiency even further. And not following the ideal engine cycles, also reduces the efficiency.
Efficiency is a valent goal to pursue, but mostly a waste of effort for a starting concern. Power to weight ratio is a much better pursuit. Think American Drag Racer. 1/4 mile per 12 gallons. 48 gpm. Excellent.
["Fool"]
["Tom Booth"]