A change of coordinates will not change the curvature of space-time but it will change the curvature of the spatial part because the division between space and time in space-time is coordinate dependent.
Again, you're failing to grasp subtleties because you haven't looked at the details.
And Newton believed in alchemy so should we stop teaching chemistry to students?
I love the irony of you telling people to read ArXiv when you ignore it when people tell you to read a 1st year beginners guide to pretty much every area of physics you open your mouth about.
Is that as far as you got?
The same can most definitely said about you.
Do you own a calendar?
When you've read anything about GR which isn't pop science perhaps you can tell people what GR says but until then at least have the decency not to pretend you understand it, particularly when talking to people who demonstrable do.
So we should pay attention to Wikipedia but you don't have to pay attention to the sum total of general relativity textbooks, papers and courses because you just know what they say, despite not having read any of them?
I really did mean space. I'm very particular about the distinction between space and space-time.James R said:
I don't misunderstand this. I'm saying the space within the universe is flat, and the universe is spherical. I note http://en.wikipedia.org/wiki/Sphere and "In higher mathematics, a careful distinction is made between the sphere (a two-dimensional spherical surface embedded in three-dimensional Euclidean space) and the ball (the three-dimensional shape consisting of a sphere and its interior)". So if you'd prefer to say it's a ball rather than a sphere that's fine by me.
We've been here before. You're dismissing Einstein. You don't understand it better than him.
And what a mistake that was. The vector approach says what it does, not what it is. It throws away the curved space.
Aaaagh! Curved space isn't the same as curved spacetime!
Yes, interpreting curved spacetime as inhomogeneous space is easy, as per Inhomogeneous Vacuum: An Alternative Interpretation of Curved Spacetime . A light beam veers like a car veers when it encounters mud at the side of the road.
Please look it up. Read what it says: The FLRW metric starts with the assumption of homogeneity and isotropy of space. Space, not spacetime.
No, that's uniform curvature. Elliptical, Euclidean, and Hyperbolic. And guess what, in Euclidean space that uniform curvature is uniformly zero. Space is flat, light rays go straight as a die.
Tell me how Euclidean space is curved.
We've already said we'll set aside the expansion of the universe. We're talking about the universe as it is now, not how it's evolved. And right now, the universe is flat. WMAP says it's flat, why do you cling to curvature in the face of the evidence and simple logic? And dismiss Einstein to boot.
Let's come back to inflation.
I'll take a look.
The time "direction" is an abstract thing. We see space and motion through it. We don't see time flowing in some direction.
The metric isn't something real that's "out there". It's a mathematical artefact associated with measurement. Space is out there. Change your motion through it and your measurements change. Inhomogeneous space affects your motion, and your measurments change again.
Einstein wasn't wrong, and nor am I. There is no gravity in homogeneous spaced.
Don't listen to AN. He attempts to spoil discussions.
They don't contradict my claim. You're in denial, prometheus.
That's a rubbish anwer. It convinces nobody.
It's a solid elastic sphere, and it's compressed. Stressed. Like a stress-ball when you let it go. It expands.
Dismissal, just like you dismissed Einstein. Don't you get it yet? When you dismissed Einstein, you dismissed GR.
You're clutching at straws and trying to hide behind a mathematical smokescreen because you've lost this argument. Read the quote again: This space-time variability of the reciprocal relations of the standards of space and time, or, perhaps, the recognition of the fact that 'empty space' in its physical relation is neither homogeneous nor isotropic". Now look up the FLRW metric as per my previous post. The FLRW metric starts with the assumption of homogeneity and isotropy of space. Space, not spacetime. Understand the difference, and that veering car. The road is straight, the surface has a gradient in mud depth, the tyre tracks are curved.
Just who do you think believes you Farsight? Do you think no one else can read the forum for themselves and see how you avoid direct questions and honest offers of discussion?
Wrong. You're conflating the views of one person with a scientific discipline. Einstein contributed a lot to many areas but in none of them is his word 'law'. He was wrong about a lot of things, as every scientist ever has been. The fact science can say "This bit of Einstein's work was wrong" without also saying "Thus we toss out all of his work" is a plus for science. As soon as you canonise someone, anyone, you go from science to dogma. Considering you're always whining about how there's entrenched dogma in the current research community your endless attempts to canonise Einstein are all the more humorous. But then I suspect its partly because of something else you said :
It wouldn't surprise me in the slightest if this betrays a subconcious association you have between yourself and Einstein. You clearly want to be hailed as 'the new Einstein' and your repeated "He and I are right, you're wrong!" hints at it further.
You keep dumping this article on us as if the title alone is supposed to prove something. Before I delve into it, here's a question: have you checked that this article actually supports your position? For example, do the authors claim they've shown a general equivalence between curved spacetime and inhomogenous space, or does it hold only under certain restricted conditions?
Misrepresentation. Nobody is "dismissing Einstein". Everyone is dismissing your interpretation of Einstein.
Nope, what we call "general relativity" today is a theory based on the principle of general covariance and the Einstein field equation:
No, he's pointing out a detail in the math, which Einstein himself stated, that contradicts your position. Calling that "clutching at straws" is disingenuous, especially when your best response:
just amounts to repeating yourself and doesn't resolve the conflict. Einstein said "...compelling us to describe its state by ten functions (the gravitation potentials gμν)", and only metrics in four dimensional spaces have ten components. This isn't going to go away; you have to deal with it. Why did Einstein speak of "ten" components and not some other number?
You say that every time someone confronts you with math. This excuse is really getting rather old. There is nothing opaque about the argument prometheus put in front of you. It is a clear, direct, succinct argument that you have failed to address, despite several opportunities now. Why did Einstein speak of ten components? It's a simple question. Can you give a straight answer?
Yes, the paper supports my position. It includes this: "The strong similarity between Eqs. (3) and (5) indicates again that an inhomogeneous vacuum may be the physical reality of the curved spacetime. From these two equations and the boundary conditions at infinity, the refractive index of this inhomogeneous vacuum can be derived as follows... Equations (6) and (7) provide a general method for finding the vacuum refractive index profile of a static spherically symmetric gravitational field, where the coefficients A(R) and B(R) can be obtained from the Schwarzschild solutions.... And remember that Einstein supports my position too. And Newton. And the scientific evidence - atomic clocks go slower in a region where gravitational potential is low. Not because of "curved spacetime", but because they employ electromagnetic phenomena. They're essentially light clocks. The clocks go slower because the light goes slower, because the vacuum impedance of space there is different to what it is at altitude.
Dishonesty? See what prometheus said here. That's a "We know better than Einstein, we can safely ignore Einstein" if ever I heard it. And he actually called Einstein an idiot, see this post.
Dismissal? What you call "general relativity" today doesn't have to bother itself with what Einstein actually said?
A retreat to your textbook? Einstein didn't talk about the curvature of space-time. He talked about the curvilinear motion of light, and the equations of motion. And the FLRW metric starts with the assumption of homogeneity and isotropy of space. Einstein said a gravitational field is inhomogeneous space. It isn't some magical mysterious action-at-a-distance. There are no gravitons. Things fall down because of the space they're in. Make space homogeneous and there's no gravitational field. Then things don't fall down. And if they don't fall down, if they don't follow curved paths and instead carry on straight as a die, because space is flat like WMAP says, where's your curved spacetime now? In the expanding universe? When at all times space is flat?
Wild accusations? It was Einstein's theory. It's consistent with experiment, as documented at http://arxiv.org/abs/gr-qc/0510072. I'm the one rooting for Einstein here, you're the guys dismissing him and calling him an idiot.
Ad hominem abuse? Just read what Einstein said, don't throw "bizarre" mud in an attempt to avoid doing so.
Disingenousness? It doesn't contradict my position at all.
Distraction?
Yes, distraction. You've totally evaded the salient points of my position and now you're attempting to discredit my argument by demanding something irrelevant. Einstein spoke of ten components because he referred to a fluid, see section 19 of The Foundation of the General Theory of Relativity. He gave what is in essence a fluid solution. In general it requires ten functions to specify a fluid.
Blandishments like this just won't wash, prometheus. You're wrong, and you still don't know the difference between curved space and curved spacetime. What are you going to do if you're in a region of curved space? So curved that it's closed? Change coordinates and hop your way out like mathematical magic? No chance.
Yep.
Get real. There's no confusion, just an attempt at cheap point scoring by playing the Hiumpty Dumpty card.
Yes. Your understanding of gravity is different to Einstein's, but you cling to your textbook teaching so much that you won't even read what he said.
They certainly aren't the same equations. They're written in a different formalism remember? You should try reading the original Maxwell sometime.
Watch my lips: you don't understand the difference between them.
You won't. You're in denial. You'll find a reason not to read it, and justify it with some lame excuse.
Really? So the curvature of Euclidean space is non-zero? Run that by me again.
Flannel. You should read more:
See that picture above? It isn't curved space. The space is flat. Homogeneous. No overall gravity. And guess what? The universe expanded even when it was very dense.
Time doesn't pass. Godel and Einstein worked it all out in 1949, see this. The passage of time is just a figure of speech. The direction of time is an abstraction. It makes as much sense as assigning a direction to all the motion in the universe, or the direction in which energy does work.
No, the Lorentz factor is derived from Pythagoras' theorem. The metric is "what you measure". It isn't something real that's out there. Nor are coordinates. You change what you measure by moving, or by moving to a place where space is different.
Stop right there. Space is fundamental, energy is fundamental. The metric isn't. It's an artefact of measurement, not "the fabric of space and time".
Wrong again. To measure distance you use the motion of light. The curvature of space-time is the gravitational field. That's a region where the speed of light varies. Hence light follows a curvilinear path. Einstein said all this, repeatedly.
The curvature of space is something that is truly real. But it isn't what a gravitational field is. A gravitational field is a curvature of space-time, and it's high time somebody else chimed in to correct you on this.
I'm not wrong prometheus. And there aren't "many counter examples already cited in this thread". If you could demonstrate why I was wrong you'd have done it by now instead of trying to bore me to death with a monster post full of ducking and diving.
He's dishonest. He won't admit it when I'm right and he won't pick you up when you're wrong, because that would be backing me up too. The guy's a thread wrecker and a serial abuser, a typical string-theory troll who patrols boards like this playing thought-police.
I can account for inflation as a time-dilation effect. There's a parallel between the expanding universe and coming out of a gravity well. It's really simple once the penny drops.
No it wasn't. Your yardsticks change, but you can't tell.
Just get a stress-ball. Squeeze it in your fist. Then let go. It's a much better analogy for the expanding universe than the balloon.
I'm the one who does understand it in the original Einstein sense. Read this to appreciate the difference between that and the modern interpretation. You don't understand GR. If you did you wouldn't be getting confused about curved space and curved spacetime. What does your job entail by the way?
See above. Pryzk's got nothing.
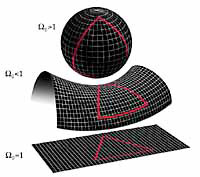
That's actually impossible, again by a simple counting argument: the gravitational field is specified by ten functions, while a refractive index is only one function. Now part of the metric is just a matter of how you pick your coordinate system, but even accounting for that you're still left with about six parameters after fixing the coordinate system, and you can't interpret six parameters as just one parameter. It's only going to be possible if the authors restrict their attention to special cases. If you check the paper this is indeed what you find. For example near the end of the first page:
Misrepresentation again. prometheus was speaking much more generally than the quote we were talking about, in saying that later generations of physicists developed a better understanding of the theory of general relativity than Einstein had. That's not really dismissing Einstein so much as building on his work.
Blasphemy!
No, why should it? If you think that by some historical mixup the theory of gravity we've been testing the last hundred years wasn't Einstein's theory, then the priority should go to the theory with the evidence, and not to Einstein just because he's Einstein.
No, a famous counter-example to your position. One you still haven't adequately addressed.
(emphasis added). I thought you cared about the distinction between space and space-time? The spatial section being flat doesn't imply that the space-time curvature is zero.
Then why is there even a debate? Take another look at the equation I posted above:
No, an ad hominem is an attack on the person making an argument rather than the argument itself. "Bizarre" was directed at the idea, not at you. Technically I suppose you could accuse me of making an appeal to ridicule for calling the idea "bizarre" without explaining what was wrong with it, though I'd have thought that should be obvious to anyone who even wants to pretend they're doing science.
You claim Einstein was talking about space and specifically not space-time. Einstein, in the same sentence, says that we need to describe "space" with ten functions $$g_{\mu\nu}$$. The metric only has ten components in four dimensional spaces. How does this not contradict your position?
Your "salient points" are an attempt at an argument from authority, a paper you only read at a superficial level, and a belief that any qualitative explanation you give for why light bends is automatically correct and the only allowable explanation.
No, he referred to $$g_{\mu\nu}$$ - the metric. And it's in your own quote: he was talking about "space", not the matter in space, which is often modelled as a fluid.
These "ten functions" are, again, the ten components of a symmetric tensor in space-time. Specifically, the ten functions referred to on your wiki page are the ten components of the fluid's stress-energy tensor. I've actually [POST=2689668]already explained this[/POST] to you.
Only if you set superficial standards for yourself and ignore everyone else's criticisms. By those standards, sure, you've "won". But in a rational debate, you don't get to dismiss something as "off-topic" or "irrelevant" just because it's inconvenient for your position.
Er he just gave an example of a difference between the curvature of space and the curvature of space-time. Incidentally, since I mentioned the appeal to ridicule in my last post, this:
is a perfect example of that particular rhetorical device.
Bloody hell you've got a paranoid take on things. How is 3 postgrads/PhDs replying to you trying to conceal this thread? We reply point by point to your posts. You refuse to reply to me, not the other way around.
Is 4 different from 2+2? They are written in different ways. Your lack of understanding of mathematical formalism is hindering you, again. Besides, the original way Maxwell wrote down his equations was using quaternions. I don't for a second think you understand quaternionic algebra. I'm not even convinced you could go well on an A Level maths exam.
Got anything other than baseless assertions? Kind of hypocritical since you complain such things exist in the mainstream.
A space-time can have Euclidean spatial components and yet have non-zero curvature. Part of your issue is you don't realise that often 'space' refers to 'space-time', since in the more mathematical approaches the underlying manifold is just called 'space', regardless of whether it refers to space, time, space-time, phase space, gauge fields etc. You should take your own advice and read a little. Well, you should read proper books, not pop science books bought from WHSmiths.
Your pictures show the spatial component, not the entire space-time. If you understood even the most basic geometry relevant to relativity, rather than working by pictures you see on Wiki, you'd know that the Minkowski space-time is flat yet non-Euclidean. It's spatial component is Euclidean but it's more than just space. The FRW metric has a similar property, you can consider the curvature of its spatial component, which is precisely what the K in the metric represents, but that doesn't mean the space-time as a whole has the same curvature.
So you're not wrong, just multiple counter examples and mistakes you've made have been provided in this and other threads, your work has been rejected from every journal you've submitted it to, you couldn't get it published, you can't provide a single model of any phenomenon, you make no quantitative testable predictions and even on forums you are laughed at.
Please provide specific examples where you have demonstrated I've been incorrect and I haven't admitted it.
That's why I offer, repeatedly, to start a new thread with you and I where we're both constrained only to reply to the topic at hand, with no insults allowed. I'm offering to discuss your work with you, along with topics like curvature, in dedicated threads where if I am rude I get a suspension.
Yes, yes, I ask you to provide a single working model of any real world phenomenon and suddenly I'm the thought police. In 1984 the thought police didn't offer to have a public discussion on the matter of disagreement, they just made you an unperson (I'm assuming you've read 1984). The refusal or restriction of public discussion is a sign of a lack of support for ones position, hence why regimes like that in 1984 avoid it. I'm offering it to you, on topics you brought up, rather than topics I might have found more interesting.
I'm afraid that isn't how it's reading, Farsight. I asked you in another thread:
Your simple counting argument is no argument at all. Einstein referred to Huygens in his foundation paper, he talks about matter conditioning the surrounding space, we employ a graded refractive index for black hole "analogies", and light bends in a gravitational field. We call this gravitational lensing. The details of how much it bends where, and how being at some location alters your measurements, does not render this paper or Einstein's own words "impossible".
This is a straw-man argument. Nobody's talking about a single parameter.
Come off it przyk. Let's find a planet and throw rocks at it. Can you see that gravitational field varying in time? No. The special case is a simple case, and it works.
It’s valid for the Schwarzschild solution and that's enough to show the principle. Particularly since the infinite time dilation at an event horizon means that as far as you and I are concerned, a black hole is static. It takes infinite time as measured by us to turn round once. So it isn't rotating.
That’s easy. We make electrons and positrons via pair production, electrons have spin angular momentum and magnetic dipole moment and can be diffracted. So you take the sphere of light approach, simplify it to a ring, then to a square. Only the horizontals bend, hence matter is affected half as much as light.
Thin gruel here. The paper supports my position.
Good. Now we're getting somewhere. Now go back and read what Einstein said about inhomgeneous space and how die Ausbreitungs-geschwindigkeit des Lichtes mit dem Orte variiert.
I’m not on my own. Other people have said this sort of thing. And I’m with Einstein. The guy who prometheus dismisses, and called an idiot.
You’re the one doing the misrepresenting. Prometheus made his opinion of Einstein perfectly clear.
Einstein said what he said.
Oh come on, pryzk, I'm with Einstein. You're not.
I’ve addressed it enough.
We’ve been through this. We said we’ll set the expansion of the universe aside. Space being flat means the universe is either infinite, or it has some kind of boundary.
Go read The Foundation of The General Theory of Relativity and show me where Einstein talks about spacetime curvature. Then read http://arxiv.org/pdf/physics/0204044, then come back to me with an argument that holds water.
This is imprecise. Einstein made a distinction between ponderable matter and matter, and said The energy of a gravitational field shall act gravitatively like any other form of energy. So think in terms of energy rather than just energy tied up as matter. When you smooth out the energy uniformly, light doesn’t bend. Think this one through. You have space with a uniform energy density, and you send a beam of light through it. Does the light bend? If so, does it curve downwards? Or up? Or to the left, or right, or some combination of the above? No. The space is homogeneous, there are no differences in any plane to make the light curve. There's no gravitational field present. It isn't just energy that results in gravity, it's a variation above the background level.
I’m quoting the guy. You're the one saying "he didn't mean that" and inserting a different meaning.
You started it. Don't do it.
A four-dimensional "space" that includes time is a mathematical space that you can't move through. We describe the metrical qualitites of space, so we aren't describing space so much as motion through it, measured in terms of distance and time.
My argument quotes Einstein, but it's evidential too. I've referred to the Shapiro delay, the GPS clock adjustment, and parallel-mirror light clocks. Light moves through space, and it moves at different speeds at different locations. And it bends because there's a gradient in the space it's moving through. It doesn't move through spacetime. It doesn't bend because it's moving through curved spacetime. Like I said, that's the effect, not the cause.
He said these confer upon space its metrical qualities.
You continue to talk of "the metric" instead of space and how you measure motion through it. I think you should examine this.
I've won, przyk. By any standards.
Clearly not the scientific standard held to by any journal, as they all rejected you.
How many refractive indices are used to describe the "inhomogenous vacuum" in the paper?
This is silly. Do you think that planets leave their gravitational fields behind as they orbit the sun? Haven't you heard of binary pulsars? We have to be able to account for non-static gravitational fields in any model that's supposed to be representative of nature.
No, this is a hasty generalization - a logical fallacy. You have given me no good reason to believe this result generalises beyond the special case the authors have considered, and I've given you at least a few reasons to believe it won't.
What are you talking about? The whole Schwarzschild solution is static. The point you're missing is that the Schwarzschild solution is only the correct solution if you're assuming the gravitational field is static and spherically symmetric. It is not a general solution. It is a special case. If the matter creating the gravitational field is not static or not spherically symmetric, the Schwarzschild solution does not apply.
Sorry, but this sounds like hand-waving. And even if I did accept that massive particles could be described as bound light states - which I don't - this behaviour is not predicted by the action principle the authors used (they just treat light as a massless point particle), so this would be another hasty generalisation. If I agreed with you, then I'd have to conclude the authors used an over-simplistic model for light, and their result still doesn't necessarily generalise beyond that.
He said:
Even if you are, what does that get you beyond an argument from authority? If Einstein said 1 + 1 = 3 would you expect us to believe it?
Where? We have homogenous but curved cosmological models that are solutions to general relativity. On what basis are you dismissing them?
Where did I agree to that?
What does this have to do with spatial curvature being different from space-time curvature?
Why don't you just tell me what $$R_{\mu\nu}$$ and $$R$$ represent, if not curvature?
This doesn't change anything: if there's energy there, there's curvature according to the EFE, no matter how homogenously it is distributed.
What, specifically, do you mean by light "bending"? As prometheus has already pointed out, this is not a good test for the presence of a gravitational field simply because the concept of "straight line" is not invariant. For example, there's a coordinate system called the Kruskal chart in which light follows straight line trajectories in the vicinity of a black hole, despite the fact there's clearly a gravitational field there. At the opposite extreme, you can get light to apparently bend even in the absence of gravity, just by accelerating or rotating on the spot.
Started what? I do not make a habit of routinely accusing people of being like creationists, or imply that they're mindlessly repeating textbooks or defending a 'special interest' just because they disagree with me. Even if I do throw in the occasional ad hominem (pretty infrequently nowadays), I only do so only after having repeatedly explained why the recipient's position is bogus, and never as a substitute for that. You, on the other hand, just implied I was mindlessly repeating a textbook (an ad hominem), and you did that instead of addressing the existence of the FRW solutions.
How does this in any way resolve the conflict it is supposed to be replying to? I'm not asking for your personal take on space-time or what the metric is. I'm confronting you with what Einstein said about them. I wouldn't say space-time was described by ten metric components anyway because the metric components are also partly a matter of which coordinate system you feel like expressing things in. But we're not discussing what you or I think. We're discussing what Einstein said. Why did Einstein say there were ten $$g_{\mu\nu}$$s?
This is all quantitative evidence for the modern theory of general relativity, which you claim isn't faithful to Einstein. Where does anyone show, quantitatively, that "gravity is inhomogenous space" theory can account for all this evidence?
Not if your aim was to convince others of your position by scientific standards. If you were just trying to convince yourself then that's too easy: you just have to cherry pick what Einstein said and turn a blind eye to the FRW solutions if you want to do that.