Photon Propagation : Straightline or Helix ?

[rpenner]

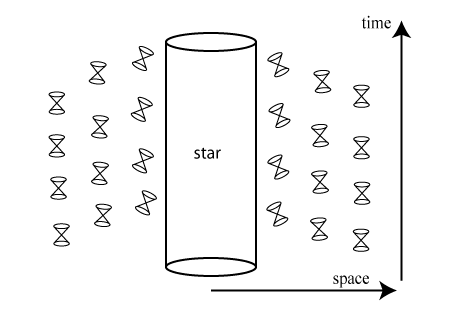
You know it, that any point motion, however weird can be considered as straightline, its a matter of unfolding the path, the way it is proposed for ant motion on a cylinder curved surface.

You are missing the point. I'm saying that depending on what the geometry of space-time is, only some motions are space-time geodesics (straight lines). Your hypothetical person at infinity, using Cartesian coordinates is making an assumption that the geometry of space-time is identical to Minkowski space and so assumes the motion of a straight line is described by $$x'' = 0, y'' = 0, z'' = 0, t'' = 0$$ when the correct equation is

$$x'' + \Gamma_{xx}^x x'^2 + 2 \Gamma_{xy}^x x' y' + 2 \Gamma_{xz}^x x' z' + 2 \Gamma_{xt}^x x' t' + \Gamma_{yy}^x y'^2 + 2 \Gamma_{yz}^x y' z' + 2 \Gamma_{yt}^x y' t' + \Gamma_{zz}^x z'^2 + 2 \Gamma_{zt}^x z' t' + \Gamma_{tt}^x t'^2 = 0$$​

where $$\Gamma_{\mu\nu}^{\rho} = \frac{1}{2} \sum_{\sigma} g^{\rho\sigma} \left( \frac{ \partial g_{\nu\sigma} }{ \partial x^{\mu} } + \frac{ \partial g_{\sigma\mu} }{ \partial x^{\nu} } - \frac{ \partial g_{\mu\nu} }{ \partial x^{\sigma} } \right) $$ is what relates geometric curvature to coordinates.

The same math that describes Minkowski space's straight lines in spherical coordinates (where $$\theta''=0$$ can't be correct, obviously) is good enough to describe straight lines in any geometry in any smooth coordinates where $$g_{\mu\nu} = g_{\nu\mu}$$ is the differentiable symmetric space-time metric and $$\sum_{\sigma} g^{\mu\sigma} g_{\sigma\nu} = \delta_{\nu}^{\mu}$$ (i.e. it has a inverse). So the equation of a geodesic line is always $$\frac{d^2 x^{\mu}}{d \lambda^2} + \sum_{\rho} \sum_{\sigma} \Gamma_{\rho \sigma}^{\mu} \frac{d x^{\rho}}{d \lambda} \frac{d x^{\sigma}}{d \lambda} = 0$$, which explains why Cartesian coordinates work great for straight line descriptions in Euclidean or Minkowski space.

 

[The God]

Rpenner,

Please refer to moons orbit around Sun......due to Earth, the orbit of moon is wavy.....you know that...Would you call it a straightline or worst would you call this wavy path as Geodesic ?? It is due to influence of earth and sun on moon, ignoring other planets etc..its no geodesic.

Below link will give you an idea about a path, which you are calling as straightline...

https://qph.is.quoracdn.net/main-qimg-8d42f754769fd0e57715cf8d01887761?convert_to_webp=true


PS : I want to shake head like krash661....

 

[paddoboy]

Now the point is
Earth's orbit around Sun can be termed as Geodesic...you can call it straightline...
Our Solar System motion around GC can also be termed as Geodesic....you can call it straightline

Bingo!
We have achieved something, albeit rather basic.
Let me state it again, The Moon, the Earth, the Sun, are all doing their best to travel in straight lines......but they do have forces acting upon them.
They consequently travel on geodesic paths.
I contend though with the best intentions, that you need to go back to those basics far more often, and then you may not make as many ignorant claims as you tend to do, nor be so inclined as to play your games [as others have noted] to disguise such lack of knowledge of the basics.
As usual, I'm glad to be of assistance.

 

[The God]

Let me state it again, The Moon, the Earth, the Sun, are all doing their best to travel in straight lines......but they do have forces acting upon them.
They consequently travel on geodesic paths.

and what are those forces ??
Some crap you write in every thread..

 

[Daecon]

Uh... gravity?

Perhaps you've heard of it...

 

[The God]

Uh... gravity?

Perhaps you've heard of it...

In GR gravity is a force !!
Is it ? Thats would be a news to many..

I wonder what all you have posted in your 2667 posts so far....

 

[rpenner]

The God has correctly faulted paddoboy for mixing-and-matching physical theories.

If one chooses to use Euclidean space and time, one is limited to a Newtonian description of gravity as a force. The exact force law becomes complicated when objects move fast or masses become large. Indeed, such schemes are bound to fail when gravity gets too strong. That's why special relativity was not good enough for gravity.

If one chooses to use General Relativity as one's description of the behavior of gravity, then at first approximation all the content is in the geometry of space-time which means satellites moves in the straightest line permissible in such a geometry. (Gravitational radiation breaks this approximation for large, fast moving objects in tight orbits.) This is a very good approximation for man-made satellites and objects smaller than moons.

But by seeking to describe curved geometry with Cartesian coordinates, The God has made the very same fault. You don't get to assume the description of straight lines in curved space-time are the same as those in flat space-time.

Would you like me to work out what the description of straight lines is in polar coordinates from first principles?

 

[Billy T]

... Would you like me to work out what the description of straight lines is in polar coordinates from first principles?

Few (none?) would follow / benefit and I know you skills with math are capable of that. Why bother?

 

[origin]

In GR gravity is a force !!
Is it ? Thats would be a news to many..

I wonder what all you have posted in your 2667 posts so far....

This post (well really the whole thread) reported. This is just more of your trolling. Clearly you just trying to antagonize everyone.

 

[Farsight]

...But by seeking to describe curved geometry with Cartesian coordinates, The God has made the very same fault. You don't get to assume the description of straight lines in curved space-time are the same as those in flat space-time.

This is somewhat misleading. Curved spacetime is associated with the tidal "force", not the "force" of gravity. See this Stanford article:

"The curvature of spacetime, then, will be depicted by the tilting of these lightcones. This reflects the fact that the causal structure of such spacetimes is different from that of flat spacetimes. So, for example, the spacetime around a massive body like a star will be depicted as follows.

Spacetime curvature corresponds to the increasing tilt of the light cones. Light curves wherever the lightcone is tilted. It doesn't follow the spacetime curvature, which is what you implied.

 

[paddoboy]

and what are those forces ??
Some crap you write in every thread..

The God has correctly faulted paddoboy for mixing-and-matching physical theories.

If one chooses to use Euclidean space and time, one is limited to a Newtonian description of gravity as a force.

Yes, certainly I accept that: The point though of the exercise and my post was in reply to the god's general misinterpretation re straight line movement and the general message I was trying to convey to the god.

But by seeking to describe curved geometry with Cartesian coordinates, The God has made the very same fault. You don't get to assume the description of straight lines in curved space-time are the same as those in flat space-time.

Yes, just one example of the many even more obvious examples of crap, personal opinions, misinterpretations and unsupported anti mainstream nonsense that he likes to flood this forum with.

 

[paddoboy]

Spacetime curvature corresponds to the increasing tilt of the light cones. Light curves wherever the lightcone is tilted. It doesn't follow the spacetime curvature, which is what you implied.

Tidal gravitational effects in no way invalidates the "light travels in geodesic paths in curved spacetime" edict. And of course the lightcone analogy is just that...a useful analogy just as is the river/waterfall model of spacetime: All do have limits of explanations and usefulness.
And of course nowhere in your Stanford link does it say that light slows or stops as per the general nonsense you like to preach to all and sundry.

Note carefully Farsight, despite how much you misinterpret the great man, GR says that energy (in its many forms like of mass, light, and whatever] tells spacetime how to bend, and the bending of spacetime tells that energy how to move. The concept of "gravity" is then exhibited in that warped/curved spacetime. The path that objects follow is called a "geodesic".

 

[paddoboy]

This post (well really the whole thread) reported. This is just more of your trolling. Clearly you just trying to antagonize everyone.

The whole crux of the matter in this and other threads in the science sections that the god has participated in, is that in his posts, he is preaching evangelistic style that the whole of 21st century cosmology is wrong, and to try and add credibility to those stupid claims, then sinks to sewer level by posting nonsense that GP-B and aLIGO were fraudulent exercises.
Most certainly bannable actions in other forums, since all of it is only his own opinion, totally and fabricated fairy tales that are unsupported, and as you say, out to antagonize those that truly are interested in real science and cosmology. That among his many other fabrications like suggesting "poster plants" to confront his nonsense [as if it is needed!] accusing me of fabricated E-Mails and then when finally confronted and warned about some of his nonsense, then starts a thread of victimization, claiming that the mods/admins are out to get him and are treating him unfairly.

The whole gist of this thread was realized in the first few replies to the OP.

I am sceptical that this post is being made in good faith. I suspect it is trying to provoke another silly argument.

Everything moves through space in a straight line unless acted upon by a force.

Your definition of straight line needs to be clarified.
[Moderator: The God is asked to respond with lengthier and better supported explanations of his reasoning, theoretical and factual basis before continuing. The God is suspected of arguing unfairly not explaining his preconceptions and/or setting up a trap and moving the goal posts when someone responds to the bait. Either would be trolling, which is forbidden by forum rules, so it is to The God's benefit to explain his position better and more fairly to his readers.]

 

[Farsight]

Note carefully Farsight, despite how much you misinterpret the great man...

I don't misinterpret Einstein, I quote him:

GR says that energy (in its many forms like of mass, light, and whatever) tells spacetime how to bend, and the bending of spacetime tells that energy how to move.

I quoted Einstein, now it's your turn. Give me a link to Einstein saying this. You won't be able to, because he never did. It's a popscience quote, and it's wrong.

The concept of "gravity" is then exhibited in that warped/curved spacetime...

The concept of "tidal force" is exhibited in that warped/curved spacetime. There's no detectable tidal force in the room you're in, and therefore no detectable spacetime curvature. But light still curves and your pencil still falls down. You can understand my point about the tilted light cones by understanding that a rolled marble follows a curved path on a tilted board. You don't need a curved board for a curved path. But as I've said previously, in this real word of energy and space, you need that spacetime curvature to get that tilt, otherwise your plot is flat and level:

But like the marble on the board, the curved path of a light beam is not following the spacetime curvature. It curves most where the slope of your plot is steepest, not where your plot is most curved.

 

[rpenner]

This is somewhat misleading. Curved spacetime is associated with the tidal "force", not the "force" of gravity. See this Stanford article:

"The curvature of spacetime, then, will be depicted by the tilting of these lightcones. This reflects the fact that the causal structure of such spacetimes is different from that of flat spacetimes. So, for example, the spacetime around a massive body like a star will be depicted as follows.

Spacetime curvature corresponds to the increasing tilt of the light cones. Light curves wherever the lightcone is tilted. It doesn't follow the spacetime curvature, which is what you implied.

As Farsight read an hour ago, that's not acceptable.

His pop-physics source is talking about curvature and geometry as being the source of gravitational phenomena. His parochial focus on "increasing tilt" ignores both that there can be no differential in "tilt" without curvature and there is no baseline of zero "tilt" without the prejudice of human aesthetics which physics has no truck with.

Curvature of space-time explains both the first-order effect of why geodesics are not Euclidean lines and the second-order effect of why neighboring geodesics don't remain parallel, with the latter giving rise to tidal forces.

I don't misinterpret Einstein, I quote him:

But did Farsight demonstrate any understanding? The quote seems generic and off topic. Is Einstein talking about the coordinate speed of light? Yes, in a quasi-Newtonian non-solution to GR.

I quoted Einstein, now it's your turn. Give me a link to Einstein saying this. You won't be able to, because he never did. It's a popscience quote, and it's wrong.

True, Einstein didn't say it. But Newton never mentioned kinetic energy which doesn't make the concept any less important in Newtonian mechanics. True, it is a pop science quote. But it is the meaning of Einstein's field equations and the geodesic equations which Einstein did publish in his scientific writing and which appear in every GR textbook. Finally, you have not argued that it is wrong in any particular way. You might come up with some ridiculously pedantic quibble but at the level it was written at it seems accurate enough.

The concept of "tidal force" is exhibited in that warped/curved spacetime. There's no detectable tidal force in the room you're in, and therefore no detectable spacetime curvature.

Ridiculous shifting of goal posts. At higher altitudes "tidal forces" are evidenced because initially parallel orbits don't remain parallel. Thus there is differential separation of geodesics.

But light still curves and your pencil still falls down.

Because, in a room-centered Cartesian coordinate system, the geodesic equations are approximately
$$ x'' = 0, y'' =0, z'' = \frac{g}{2c^2}, t''=0$$
These are derived from the geodesic equation so it's a fairly simple exercise in coordinate transformation to derive more precise values.

You can understand my point about the tilted light cones by understanding that a rolled marble follows a curved path on a tilted board. You don't need a curved board for a curved path.

Farsight's analogy appears to be using gravity to explain gravity. That's pretty useless.

But as I've said previously, in this real word of energy and space, you need that spacetime curvature to get that tilt, otherwise your plot is flat and level:

But like the marble on the board, the curved path of a light beam is not following the spacetime curvature. It curves most where the slope of your plot is steepest, not where your plot is most curved.

That's a math claim, but I see no math.

 

[paddoboy]

I quoted Einstein, now it's your turn. Give me a link to Einstein saying this. You won't be able to, because he never did. It's a popscience quote, and it's wrong.

I didn't say Einstein said it....I said that GR says it.
And from memory it is a John Wheeler quote, the exact words going along the lines of "Spacetime tells matter how to move; matter tells spacetime how to curve."
Just to add, Einstein as great as he was, also was wrong on occasion: To his great credit though, he was humble enough to admit when in error> Perhaps you and a few others need to take a page out of his book, instead of misquoting and taking phrases of his out of context.

 

[paddoboy]

True, it is a pop science quote. But it is the meaning of Einstein's field equations and the geodesic equations which Einstein did publish in his scientific writing and which appear in every GR textbook. Finally, you have not argued that it is wrong in any particular way. You might come up with some ridiculously pedantic quibble but at the level it was written at it seems accurate enough.
.

Bingo!
Just because any "pop science" aspect is quoted, does not mean its wrong.
This seems to be a common ploy or cop out among those that do not seem to recognise the standard accepted mainstream picture.
https://en.wikipedia.org/wiki/Popular_science
Popular science is a bridge between scientific literature as a professional medium of scientific research, and the realms of popular political and cultural discourse. The goal of the genre is often to capture the methods and accuracy of science, while making the language more accessible.
The purpose of scientific literature is to inform and persuade peers as to the validity of observations and conclusions and the forensicefficacy of methods. Popular science attempts to inform and convince scientific outsiders (sometimes along with scientists in other fields) of the significance of data and conclusions and to celebrate the results.
""""""""""""""""""""""""""""""""""""""""""""""""""""""""""""""""
While certainly some "pop science" stuff can be wrong, in most of those cases it can be attributed to poor journalism.eg: The headlines from physorg a year or so ago went along the lines of "Hawking says BH's do not exist"
which on face value was entirely wrong but did eventually note in the article that all that was inferred was the nature of information lost, firewalls and quantum theoretical applications, which in no way did invalidate BH's.

 

[The God]

Curvature of space-time explains both the first-order effect of why geodesics are not Euclidean lines and the second-order effect of why neighboring geodesics don't remain parallel, with the latter giving rise to tidal forces.

Rpenner,

I posted a link in my post # 42, which gives the motion profile of Moon around Sun, you are yet to respond how that is a straightline (geodesic) in GR.

On your above assertion, please note that two photons (travelling on geodesic, null geodesics) initially parallel, will remain parallel and will not converge, so that contradicts your above statement.

You also would like to confirm if the resultant path (a single object path due to multiple sources) is also actually a geodesic in GR ?

 

[rpenner]

Rpenner,

I posted a link in my post # 42, which gives the motion profile of Moon around Sun, you are yet to respond how that is a straightline (geodesic) in GR.

That answer was given in detail in post #41. The detail requested is necessarily mathematical as was the detail given.

On your above assertion, please note that two photons (travelling on geodesic, null geodesics) initially parallel, will remain parallel and will not converge,

That does not follow. If $$T^{\mu}$$ is the direction of a null geodesic through an event. And $$X^{\mu}$$ is the direction of separation between initially parallel geodesics, then we have them staying parallel if

$$R_{\nu\sigma\tau}^{\mu} T^{\nu} T^{\sigma} X^{\tau} = 0$$ which is necessarily unlikely if the Riemann (mixed) curvature tensor, $$R_{\nu\sigma\tau}^{\mu}$$ is not zero.

so that contradicts your above statement.

Consequently you have not demonstrated a contradiction. It will be impossible to tackle that task in good faith without learning the required math and physics of General Relativity.

You also would like to confirm if the resultant path (a single object path due to multiple sources) is also actually a geodesic in GR ?

Yes, that's confirmed. Because the trajectory is given by the geodesic equation.

 

[The God]

That answer was given in detail in post #41. The detail requested is necessarily mathematical as was the detail given.

That does not follow. If $$T^{\mu}$$ is the direction of a null geodesic through an event. And $$X^{\mu}$$ is the direction of separation between initially parallel geodesics, then we have them staying parallel if

$$R_{\nu\sigma\tau}^{\mu} T^{\nu} T^{\sigma} X^{\tau} = 0$$ which is necessarily unlikely if the Riemann (mixed) curvature tensor, $$R_{\nu\sigma\tau}^{\mu}$$ is not zero.

Consequently you have not demonstrated a contradiction. It will be impossible to tackle that task in good faith without learning the required math and physics of General Relativity.

Yes, that's confirmed. Because the trajectory is given by the geodesic equation.

1. So in principle you are saying that two photons, initially prallel will not remain parallel.

2. Secondly what you are saying, that any particle path, even in presence of multi sources (of gravitation), shall becalled as straightline ? The corollary question is resultant of geodesics is also geodesic ?

If so, we introduce an external force on that particle, now by definition that particle would be on non geodesic, so it shall be termed as non-straightline ?