Absolutely not. Regardless of the problems GR produces for us in defining "now", I think we can all agree that any definition of it which connects two time-like separated points would be a poor one. Do you disagree with me on this?
No, I don't disagree. (Or yes, any definition that calls two timelike separated points "now" would be a bad one.)
Because you can change labels all you want, including the definition of time-like separation to be compatible with Kruskal coordinates, but most humans appreciate the difference between yesterday and tomorrow.
Space- and timelike separation are not defined in terms of coordinates. They never were in GR. There is no redefinition or reinterpretation taking place whatsoever. In GR, space- and timelike separation are and always have been defined in terms of the (invariant)
spacetime interval. You can practically read straight off the
Schwarzschild metric that the Schwarzschild $$t$$ and $$r$$ coordinates are
not timelike and spacelike, respectively, everywhere. For instance, take two infinitesimally separated events of Schwarzschild coordinates $$(t,\,r)$$ and $$(t,\, r \,+\, \mathrm{d}r)$$, occurring at the same Schwarzschild coordinate time $$t$$. The expression of the Schwarzschild metric tells you that the spacetime interval between these events is
$$\mathrm{d}s^{2} \,=\, (1 \,-\, r_{\mathrm{s}} / r)^{-1} \, \mathrm{d}r^{2} \,.$$
This is positive when $$r \,>\, r_{\mathrm{s}}$$ (outside the event horizon), in which case the two events are spacelike separated, and negative when $$r \,<\, r_{\mathrm{s}}$$ (inside the event horizon), in which case GR is telling you that these two events, with the
same $$t$$ coordinate, are actually
timelike separated.
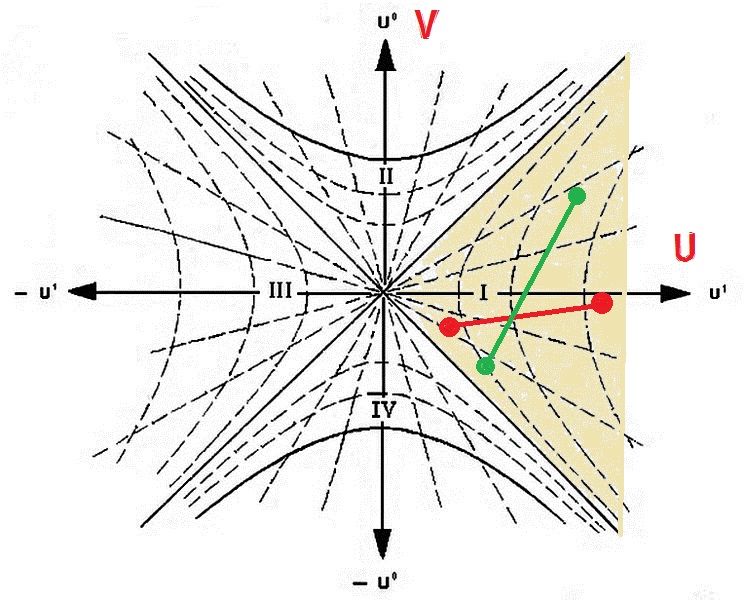
The claim that the Kruskal chart should suffice in proving the existence of black holes today connects our points in spacetime with one which is time-like separated from us.
The Kruskal chart
should suffice, for someone who actually understands GR, because it is deliberately defined in such a way as to make causal relations very easy to visualise. Usually you have to calculate $$\mathrm{d}s^{2}$$ from the metric expression to see if two events are space- or timelike separated in GR (and at all events along a curve, if the two events aren't infinitesimally separated). But in Kruskal coordinates it's easy: they're defined in such a way that the metric expression takes the form
$$\mathrm{d}s^{2} \,=\, f(v,\, u)^{2} \bigl( -\, \mathrm{d}v^{2} \,+\, \mathrm{d}u^{2} \bigr) \,,$$
so telling whether two events are space- or timelike separated is a simple matter of looking at whether $$- \, \Delta v^{2} \,+\, \Delta u^{2}$$ is positive or negative in Kruskal coordinates, even for finitely separated events.
See? No interpreting or trying to be clever or anything here. This is just taking a very basic definition from GR and applying it.