It isn't very heavily coordinate dependent.
This is wrong. Gravitational time dilation is more or less associated with the $$g_{tt}$$ metric component. (If there's a more formal definition of what gravitational time dilation is and how it should be predicted from the theory, I've certainly never seen it.) The metric components $$g_{\mu\nu}$$ are explicitly coordinate dependent. This is one of the absolute most basic things to understand about general relativity.
I've already given a well-known example where this is clearly visible: accelerating reference frames. The gravitational time dilation factor from the perspective of an observer in SR can be constant (one), finite, infinite, or even negative depending on whether or not the observer is accelerating, and at what rate. You never address this.
Observers see the lower clocks going slower, and the meaning of the infinite time dilation is the whole point of our discussion.
You are confusing gravitational time dilation (a coordinate-dependent quantity) with two
different quantities that are actually observable:
1) The
apparent slowing of one clock as seen by a distant observer looking at it through a telescope (this is the relative Doppler shift factor).
2) The accumulated times shown on two clocks when they're brought side-by-side together for comparison. Simultaneity is only well defined for two events at the same place and at the same time in relativity, and the times shown on two clocks at the same place is the only invariant comparison that can be made.
These are what are
actually measured in experiments. As far as what the theory has to say about them, the metric in Schwarzschild coordinates has the nice feature that it's static. This makes it very convenient for working out the Doppler shifts and accumulated times between two clocks
if they spend a substantial amount of time at fixed Schwarzschild radii. This doesn't generalise, though.
Please tell me more about this negative gravitational time dilation.
There's a standard way of defining an accelerating observer's reference frame, which I described in some detail in a [POST=3051585]previous post[/POST]. (It's the same thing as the
Rindler coordinate system, except that I put the accelerating observer at $$x \,=\, 0$$). In units where $$c \,=\, 1$$, the proper time element in the accelerating coordinate system works out to be $$\mathrm{d}\tau^{2} \,=\, (1 \,+\, ax)^{2} \, \mathrm{d}t^{2} \,-\, \mathrm{d}x^{2}$$, where $$a$$ is the acceleration felt by the observer at $$x \,=\, 0$$. For a clock at some fixed $$x$$ coordinate, you find from this that the accumulated proper and coordinate times are related by
$$\mathrm{d}\tau \,=\, (1 \,+\, ax) \, \mathrm{d}t \,.$$
To relate this to something you might have seen before, you might like to compare this with the analogous formula for gravitational time dilation in a weak gravitational field: $$\mathrm{d}\tau \,=\, (1 \,+\, \Phi/c^{2}) \, \mathrm{d}t$$, where the Newtonian gravitational potential $$\Phi \,\approx\, gh$$ if the field is roughly constant.
Obviously, $$1 \,+\, ax$$ becomes zero (infinite gravitational time dilation) at the location $$x \,=\, -\,1/a$$, and negative (so negative time dilation) for $$x \,<\, -\,1/a$$.
This is easy to understand as a consequence of the relativity of simultaneity effect. As you hopefully already know, if two events (say two explosions called A and B) occur with a spacelike separation in space and time, then their order (which happens first) is frame-dependent. There are reference frames in which A happens before B, there are reference frames in which they happen at the same time, and there are reference frames in which B happens before A. So if an explosion happened in space 10 light years away and 1 second ago from your point of view, you could quickly accelerate to a different velocity, and the explosion could still be 1 second in your
future in your new frame. Negative gravitational time dilation is just this expressed during the period of acceleration.
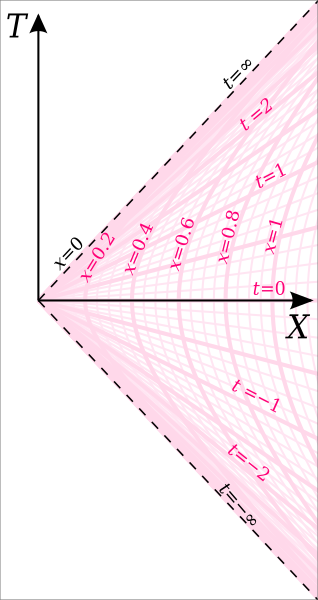
If you're familiar with Minkowski diagrams, it's also easy to see what's happening. Borrowing Wikipedia's diagram for the Rindler coordinate chart, the situation looks like this:
Basically, things work out in such a way that the accelerating observer's $$x$$ axis (represented by the $$t \,=\, 0,\,1,\,2,\,\ldots$$) always crosses the origin of the Minkowski diagram, and it starts to sweep
backward on the left-hand side as you trace along the accelerating observer's worldline.
Of course,
none of this is directly measurable, and the remedy is simple: you recognise it as a harmless artefact of defining accelerating reference frames in this particular way. The moral I'd encourage you to take from this is that even seemingly "natural" and obvious coordinate systems can exhibit some very strange behaviour, without it actually representing anything physical. That's why you should take it with a grain of salt when the Schwarzschild metric includes things like infinite gravitational time dilation and zero coordinate speed of light: accelerating frames also exhibit these features, and I'm sure you'd agree it doesn't really mean anything in that case.
The light path lengths are the same. Hence the invariant interval.
No, that's not what the spacetime interval is or why it's invariant. The spacetime interval is a quantity that's measurable in the following sense: if you have two infinitesimally spacelike separated events located at coordinates $$x^{\mu}$$ and $$x^{\mu} \,+\, \mathrm{d}x^{\mu}$$, then there's a locally inertial reference frame in which they occur simultaneously. In that reference frame, the spacetime interval is the same thing as the distance between the events that you'd measure using a ruler.
The way to see this is to notice that in a locally inertial reference frame, "simultaneous" means $$\mathrm{d}t \,=\, 0$$, in which case the spacetime interval just reduces to Pythagoras' theorem: $$\mathrm{d}s^{2} \,=\, \mathrm{d}x^{2} \,+\, \mathrm{d}y^{2} \,+\, \mathrm{d}z^{2}$$.
It isn't misleading when you understand it. Now please read the
time travel is science fiction OP and concur with it. It shouldn't be a problem. Then we can take the next step. We need to do this to avoid going round in circles.
I'm already familiar with your views on time travel from previous threads. Basically you're saying time travel is impossible because it would contradict what you believe about time. I don't think that's a good argument. You're not considering the possibility that your beliefs about time might turn out to be wrong. There's nothing wrong with saying there's no evidence for time travel, or that there's no theoretical basis for it in mainstream physics, or that there are paradoxes that seem to make the idea problematic. That's fine. Time travel is consigned to our science fiction stories at the moment, and there's good reason to believe it will stay that way. But you seem to want to ban even "what if"
speculation on the subject, and I don't agree with that attitude.
You also neglected to mention the only two modes of time travel I'd actually be torn to make a choice between: flying DeLorean vs. 1960s-style blue police box.