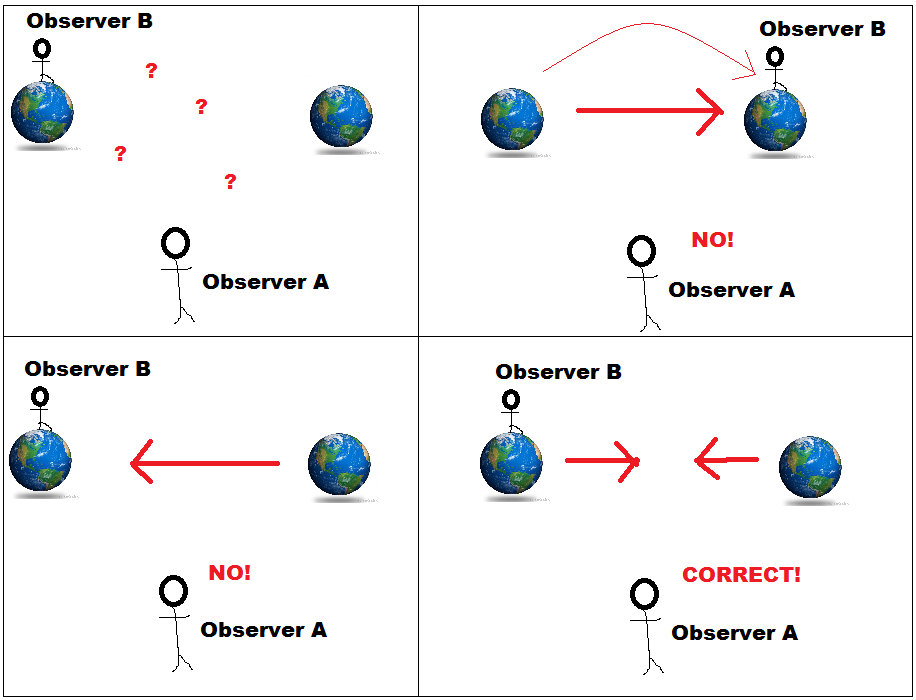
Dammit paddoboy, you are right if the Earth is NOT AFFECTED BY THE ACCELERATION. That is the critical assumption by Galileo, et al, because it's such a small factor when we're discussing small objects. Use the following equationThe heavier item may have a greater attraction but it also has great resistance to change...Intertia:
The lighter item has less attraction, and also less Inertia and less resistance to change/movement/falling.
So we have one item with great gravitational attraction and more intertia, fighting that attraction...and the other have less gravitational attraction but also less Inertia fighting the gravitational pull.
Guess what? The two effects balance each other out, so that they hit the ground at the same time.
Galileo was right!
t = sqrt(2d/(a_o.e+a_e.o))
but consider a_e.o (the acceleration of Earth towards the object) t0 be ZERO. That equation will give you the same answer regardless of what mass the object is INCLUDING A BLACK HOLE!
You are blindly hoping that Earth moving towards the object somehow cancels out but you're not exactly sure how. I'm explicitly showing you the math to do so, and the math shows that you are mistaken. Period.