I want you to talk us through it and say what each term means. What's the problem?
No problem.
The first summation expresses the electric field in a particular basis. The second sum is the magnetic field. The circle with the cross in it is an operator for the outer product between the two sums. On the right-hand side we have the tensor components in terms of the tensor product formed by the outer product.
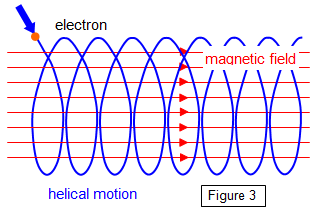
You said the arrowheads denoted the direction of the field rather than the force. But you can't explain why the "inward-field" electrons and the "outward-field" positrons repel each other.
Sure I can. When a charged particle is placed in a field (caused by one or more other charged particles), that charge experiences a force in the direction of the net field if the charge is positive, and a force in the opposite direction if the charge is negative.
But I thought I'd already explained this to you mathematically in my previous post. Didn't you understand?
And we've now established that the field is Fμv and it doesn't point in some direction. The arrowheads don't denote the direction of the field. There is no inward field or outward field. The electron and the positron have opposite chiralities.
I think that because you don't know what a tensor is, you don't understand why it doesn't "point" like a vector does. When you talk about "arrowheads", I assume you're talking about the ones on the field diagram. Those are like electric field vectors, and they do point. There is an inward or outward field depending on which direction those arrows point. You even provided the diagrams yourself. As for "chirality", I doubt you know what that term refers to.
Well it isn't true. The electron's field is the electromagnetic field.
I already walked you through this. An electron has only an electric field when it is stationary. If it moves, it has an electric and a magnetic field. Call that the electromagnetic field if you want to.
It doesn't change just because you moved.
Yes it does. That's relativity.
Let's just say that you're holding an electron in your hand, and I'm holding another. When your electron is motionless with respect to my electron, you can feel a linear force pushing you away. You say you're in an electric field. Then when you walk past me you also feel your electron swirling in your hand. Then you say you're in a magnetic field too.
No. If you remain stationary, there's no magnetic field from your electron. Only moving charges create magnetic fields. Also, I don't get what you mean by I feel the electron "swirling". What does that mean? Magnetic forces aren't "swirly" forces. They point in straight lines, just like other forces. Forces are vectors quantities.
I know why. Electrons and positrons are spin ½ particles, and they have the opposite chirality.
Define "chirality" for me, please.
Yes it is. Because the vector points both ways.
Your assertion that the field of a single charge points "both ways" is just wrong. I don't know where you get this stuff.
Now we're getting somewhere. The field is the electromagnetic field. It doesn't point inward, or outward.
An electromagnetic field is just electric and magnetic fields, nothing more. Of course, you can have the electric part pointing one way and the magnetic part pointing another way. Suppose they are at right angles, as they are in an electromagnetic wave. Tell me, then, Farsight. Which way does the "electromagnetic" field then point, and how do you work it out? Please show me the maths, too.
Strictly speaking, it doesn't really point in any direction. But since North is a whole host of directions and we can understand North, we can also understand the "direction" of the electromagnetic field. It's the same as the direction that this thing is spinning:
That's a pretty picture, but it doesn't tell us anything about electromagnetic fields.